Textural Equilibration
Textural equilibration generally starts at grain boundaries, and in particular at three-grain junctions where the dihedral angle adjusts to the equilibrium value. This sets up a change in curvature near the junction that propagates outwards (Figure 13), creating a surface of constant mean curvature along the entire interface (Voll, 1960). Once the grains have attained their minimum energy shape (which includes a certain area of planar faces due to the inherent anisotropy of most rock-forming minerals, e.g. Figure 1b), the microstructure will then evolve towards a unimodal grain size by coarsening (Higgins, 2010). The mechanisms by which this equilibration process occurs are diffusive, and therefore occur more rapidly at higher temperatures (Holness et al., 1991) and in finer-grained rocks. Equilibration is also faster in the presence of fluid phase, either H2O (Holness et al., 1991) or a silicate melt. The effect of H2O in enhancing diffusion, even in vanishingly small concentrations (<<0.1 wt. %) at which the H2O molecules are likely to be adsorbed on grain boundaries rather than forming a free phase, is well known, with major effects on metamorphic reaction textures. The presence of H2O may also enhance rates of grain boundary migration (Schenk and Urai, 2004). Mass transport in melt-filled pockets is higher than on dry grain boundaries, so melt-filled pores reach equilibrium relatively rapidly.
Most rocks with well-equilibrated microstructures are granulites (Figure 9f) and hornfelses. Textural equilibrium is also commonly seen in mono-mineralic rocks at lower grades such as carbonates and quartzites (e.g. Figure 1a). This is because it is much easier to re-organise grain boundaries by movement of material across them (only possible if the two grains on either side are of the same phase) compared to moving material along them (necessary for inter-phase boundaries). The requirement for grain boundary diffusion is also the reason why fine-grained poly-phase aggregates equilibrate faster than their coarse-grained equivalents .
Because the process of equilibration is diffusive, the critical parameter for equilibration is the time-integrated thermal history. Thus rocks need to be held at sufficiently high temperatures (in the absence of reaction or deformation) for a long time. This means that while a gabbroic cumulate may solidify at temperatures well above 1100˚C it is possible that the augite-plagioclase-plagioclase dihedral angles change little from those originally inherited from the original solidification, whereas a granulite may be completely texturally equilibrated despite not having been heated above 900˚C. The critical difference between the two is the period over which they remained hot. The granulite may have been at high temperatures for 106 years, while typical km-scale crustal gabbroic intrusions cool to ambient temperatures over 105 years.
Figure 13. Dihedral angles are the first sign of textural modification
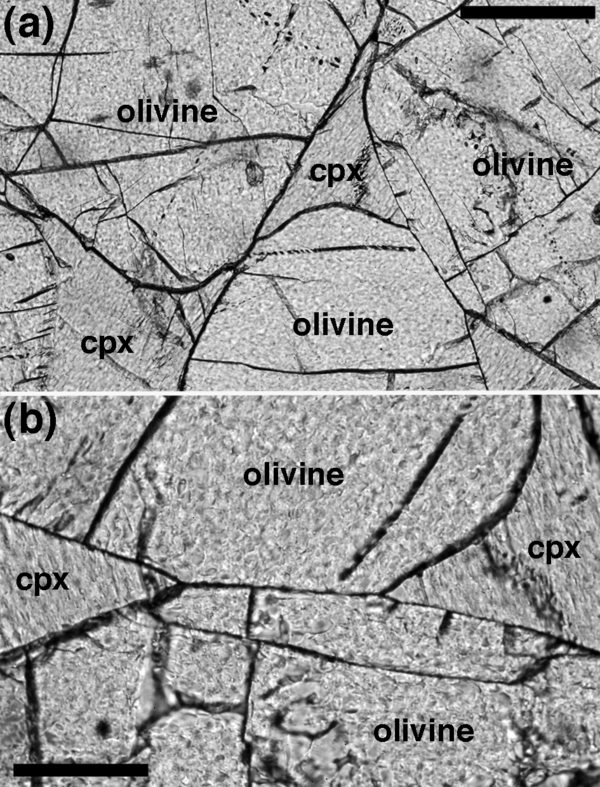
Cumulus olivine grains enclosed by an augite oikocryst. Eastern Layered Intrusion, Rum. (a) Note how the dihedral angle is larger than expected from extrapolation of the curved interfaces far from the junction itself. Scale bar is 200 μm long. A pair of augite-olivine-olivine junctions from (a) is enlarged in (b). Scale bar is 100 μm long. The dihedral angles in this oikocryst were analysed by Holness et al. (2005a) and this photomicrograph shows a central part of the oikocryst.
A useful concept, used extensively for diffusive processes (e.g. re-setting of isotopic ratios), is the closure temperature – that temperature at which mass transfer by diffusion becomes so slow that it effectively ceases to make significant differences to the microstructure. In a similar way to that for isotope ratios, the closure temperature is sensitive to the cooling rate. Rocks that are rapidly cooled have a higher closure temperature for microstructural change than rocks that were more slowly cooled. This means that it is possible to quench relatively unmodified microstructures in a km-scale gabbro body intruded into the shallow crust, while a rock that reached a lower maximum temperature may approach textural equilibrium more closely.
A measure of the closure temperature, and an indication of the timescales for dihedral angle modification, is provided by Holness et al. (2005a). The augite-olivine-olivine- dihedral angles in a cluster of three contiguous augite oikocrysts in a peridotite from the Rum Eastern Layered Intrusion decrease from the centres to the edges of the oikocryst. The population of angles around the edges of the oikocryst is similar to that observed in texturally equilibrated melt-bearing olivine aggregates, while that in the centre is closer to that expected for equilibrated augite-olivine-olivine junctions. Holness et al. (2005a) interpret the data as an indication that the augite-olivine-olivine angle inherited an equilibrated melt-olivine-olivine angle that then was modified towards the higher angles expected for augite-olivine-olivine equilibrium. That the angle changes from the centres to the edges demonstrates not only that this process was occurring during the growth of the oikocryst itself, but that it effectively stopped during the last stages of oikocryst growth: the closure temperature for dihedral angle change was therefore close to that at which the oikocryst stopped growing, and may have been of the order 1000˚C.
A similarly high closure temperature is indicated by Harte et al. (1993) who report bimodal populations of ilmenite-olivine-olivine angles in the Matsuko peridotites (a suite of xenoliths entrained by a kimberlite). The low angles are interpreted as an inheritance from a melt-bearing precursor. Although we don’t know the precise history of these xenoliths their mantle source is indicative of high temperatures and thus a high closure temperature for dihedral angle modification.
Case study: The variation of dihedral angles in layered intrusions
An example of information which can be obtained from a detailed examination of dihedral angles in non-equilibrated rocks is provided by the body of work done on layered gabbroic intrusions by M.B. Holness and co-workers. This work concentrates on the augite-plagioclase-plagioclase angle because three-grain junctions between these two phases tend to be fresh and unaltered (in contrast to those involving olivine) and because these two phases occur throughout many layered mafic intrusions, even those in which fractionation has reached the extreme endpoint (e.g. Skaergaard). Most of the published work is based on comparisons of the median value of the population of augite-plagioclase-plagioclase dihedral angles, Θcpp. The value of Θcpp lies between the original value inherited during solidification from an impingement texture (60 – 70˚) and that pertaining to a texturally equilibrated solid (114˚).
Within a single intrusion, Θcpp correlates with the liquidus assemblage of the cumulate (Holness et al., 2007a, 2007c). In the Skaergaard intrusion, comprising a continuous succession of increasingly fractionated floor cumulates, Θcpp increases in a step-wise fashion at the arrival of each of the cumulus phases augite, Fe-Ti oxides and apatite (Holness et al., 2007a; 2007c). The Rum Eastern Layered Series comprises an alternating series of olivine cumulates and plagioclase-bearing cumulates on a 1-50m scale (Emeleus et al., 1996). The latter form layers up to 40m thick of either gabbros (with cumulus plagioclase, olivine and augite) or troctolites (with only plagioclase and olivine as cumulus phases). The value of Θcpp in the troctolites is ~ 80˚, while that in the gabbros is ~90˚ (Holness, 2005; Holness et al., 2007c).
At first sight one might suspect that the dihedral angles at augite-plagioclase junctions in mafic cumulates have a compositional control. However, that the control is primarily thermal is illustrated by the troctolitic cumulates underlying an intrusive peridotite sill in the Eastern Layered Intrusion of Rum (Holness, 2005). The Θcpp values in the troctolite increase over a few metres towards the peridotite body, from ~ 80˚ to ~100˚. The mineral assemblage in the troctolite remains the same, so the increase in angle must be a consequence of the greater opportunity for textural equilibration in the aureole of the peridotite sill, in a similar fashion to the varying angle population through a single oikocryst.
The step-wise changes in Θcpp at the arrival of a new liquidus phase in Skaergaard, and the bimodal Θcpp in the alternating Rum cumulates have been explained using the concept of fractional latent heat (Holness et al., 2007a). The loss of heat from a body of magma is controlled by conduction through the walls, roof and floor. However, in the temperature interval over which crystallisation occurs, there are two sources of heat contributing to the enthalpy budget: the sensible heat (the actual temperature of the body) and the latent heat of crystallisation. The fraction of the enthalpy budget that is provided by latent heat is known as the fractional latent heat, and this increases through the crystallization interval, reaching unity at the terminal eutectic point (Holness et al., 2009). The increase in fractional latent heat is step-wise at the arrival of each new phase on the liquidus. Holness et al. (2007a) suggest that the step-wise increases in Θcpp reflect this change in fractional latent heat, due to a corresponding decrease in the rate at which the sensible heat falls during crystallization. The cumulates keep hotter for longer, thus permitting an increase in the dihedral angle.