Scaling

For analogue models to give answers to geological questions, the models must be similar to nature in various respects. The similarity should not only be geometric, but kinematic and dynamic similarity must also be considered. This concept was first applied to geology
by Hubbert (1937) who quoted Galileo Galilei:

Clearly if then one wishes to maintain in a great giant the same proportion of limb as that found in an ordinary man he must either find a harder and stronger material for making the bones, or he must admit a diminution of strength in comparison with men of medium stature; for if his height be increased inordinately he will fall and be crushed under his own weight. Whereas, if the size of a body be diminished, the strength of that body is not diminished in the same proportion; indeed the smaller the body the greater its relative strength. Thus a small dog could probably carry on his back two or three dogs of his own size; but I believe that a horse could not carry even one of his own size.

As the mass of a body and the strength of its bones scale with the cube and the square of the length, respectively, the material of the bones needs to be strengthened proportionally to the scaling of length. Accordingly, even early "analogue" experiments performed in the 19th century (see Figure 2, reproduced from Cadell 1889) used materials with properties different from those of the natural prototypes. In these attempts to explain geological processes the scaling was done rather intuitively than mathematically.

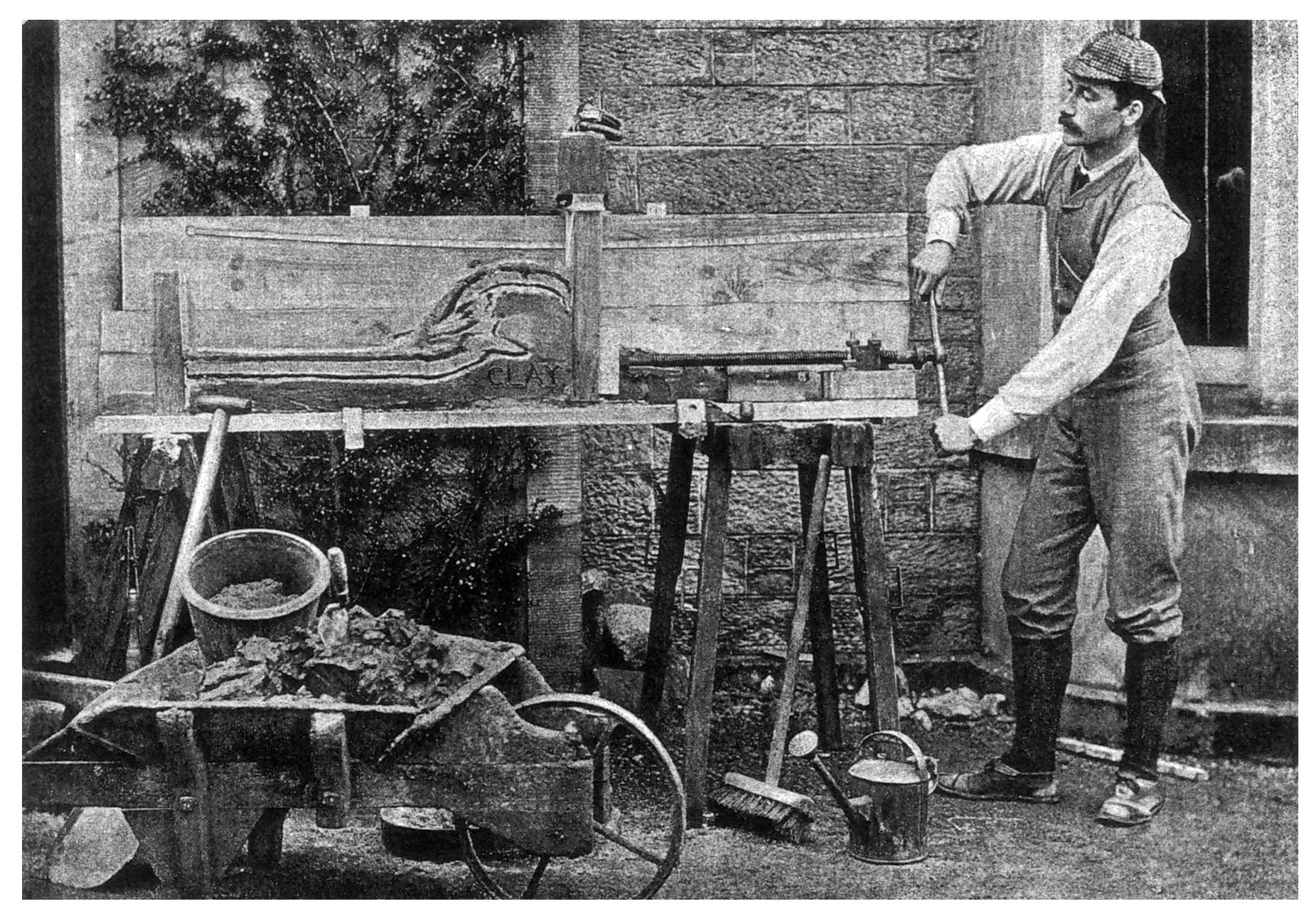Figure 2. Early analogue modelling done by Cadell (1889). No scaling was done for this experiment, concerned with the generation of the Scottish Highlands. (Click for enlargement)

Similarities

Similarities between models and nature are rooted in processes which the model is supposed to reproduce. The physical laws governing these processes can be expressed as equations that can be used to link various properties of the model with corresponding properties in nature.

Geometric Similarity. Geometric shapes are considered to be "similar" if corresponding angles are equal. This implies that all possible ratios of lengths in the model must be the same as the corresponding ratios in nature, e. g. the aspect ratio. Exaggeration of the vertical scale does not maintain the aspect ratio. For geometric similarity all lengths must be scaled by the same scaling factor. Angles in the model must equal those in nature.

Kinematic Similarity. A prerequisite for kinematic similarity is geometric similarity. Furthermore, all the time needed for corresponding movements must be scaled by the same factor. Therefore, scaling factors for velocity, strain rate and other kinematic properties are also set by this constraint to maintain kinematic similarity.

Dynamic Similarity. Dynamic similarity deals with accelerations, forces and related properties. Therefore, the masses of corresponding volume elements dV must all be scaled by the same factor, i. e. density must be scaled homogeneously throughout the model. The relevant types of forces are:

Body Forces. Body forces act on the mass of a body. Since the density scale is given, gravitational forces F = mg are only influenced by gravity g. Inertial forces can be neglected in tectonic models. Displacement rates in tectonics are of the order of some centimetres per year. To result in accelerations comparable to g, the velocities would have to be changed in the order of nanoseconds.

Surface Forces/Stresses. Forces acting on surfaces (stresses) require geometric similarity. Without this condition being fulfilled, the scaling of surface forces depends on the angle between the force and the surface normal. Stresses are to be preferred to surface forces because stresses are intensive properties.

Viscous Stresses. Instead of viscous forces, their intensive equivalent, viscous stresses should be used. These build up in a material being deformed by flowing. In order to obtain dynamic similarity with respect to viscous stresses, the viscosity must be scaled. As the viscosity of the materials in question (waxes in the model, rocks in nature) depends on temperature, the temperature dependency of the model materials must be similar to that in nature.

Thermal Similarity. In order to obtain thermal similarity, various factors have to be taken into account:

Kinematics. Kinematic similarity requires the scaling of times needed for corresponding mechanical movements. Consequently, thermal relaxation times must also be scaled. Thus, advective heat transfer (governed by mechanical movement) and conductive heat transfer (governed by thermal diffusion) are treated equally.

Rheology. To obtain dynamic similarity with respect to viscous stresses, the temperature-dependent rheology in the model needs to follow the same type of relationship as in nature. This leads to a scaling factor for the activation energy.

Energy. In general, temperature-related properties referring to thermal energy such as thermal conductivity or heat flux must be scaled as well. Nevertheless, if thermal energies do not contribute to the deformation, this demand can be neglected.

The concept of similarities leads to three different approaches which are mathematically equivalent.

Dimensionless Numbers One way to verify similarities is to use dimensionless numbers, i. e. ratios of properties sharing the same dimension within one system. Equality of the dimensionless numbers then ensures similarity between model and nature. Using dimensionless numbers, several systems can easily be compared quantitatively (Ramberg, 1967; Ranalli, 1987).

Non-Dimensionalisation Instead of using ratios of properties sharing the same dimension, the concept of non-dimensionalisation uses characteristic values of any property to obtain dimensionless values (Weijermars & Schmeling, 1986). This extension of the concept of dimensionless numbers requires several equations linking the characteristic values to be fulfilled.

Scaling Factors In this work, ratios between corresponding values in the model and in nature ("scaling factors") are used. Some of these scaling factors can be chosen arbitrarily, others are set by equations linking the scaling factors. This concept offers large flexibility, and inconsistencies in the scaling are easily visualized (Hubbert, 1937; Ramberg, 1967). The next section describes the scaling factors as well as the equations linking them.